When I was in high school, I didn’t think mathematics was particularly interesting. To be honest, it seemed so easy at first that I studied it for the first time after entering university. However, during my first math lecture as an undergraduate, I realized that everything I thought I knew about math was wrong. It was never easy. I quickly discovered that mathematics can be really exciting, especially when you go beyond the realm of pure arithmetic.
In physics, some truly surprising content, counterintuitive concepts about the universe, emerge in high school. At this time, students can get a glimpse of the strange quantum world and encounter Einstein’s theory of general and special relativity. School mathematics cannot keep up with these wonders. Learn basic arithmetic operations, integration and derivation, and basic processing of probability and vectors. If you’re lucky, an ambitious teacher might teach you a simple proof. That’s it. Therefore, it is no wonder that many students fail to develop a true passion for this subject.
But mathematics offers all sorts of surprises, like the Banach-Tarski paradox that a sphere can almost magically double, or the fact that there are infinitely many different infinities. What really surprised me was discovering how deeply mathematics is intertwined with the strangest physical phenomena. It’s not necessarily quantum physics itself that produces surprising effects. No, the system always follows the strict rules of mathematics. Chemist Peter Atkins wrote in his 2003 book: galileo’s finger“Determining where mathematics ends and science begins is as difficult and pointless as mapping the edge of morning fog.”
About supporting science journalism
If you enjoyed this article, please consider supporting our award-winning journalism. Currently subscribing. By subscribing, you help ensure future generations of influential stories about the discoveries and ideas that shape the world today.
Few examples illustrate the blending of mathematics and physics better than the discoveries made by physicist Michael Berry. In 1984, Berry uncovered a profound and largely unexpected geometrical aspect of quantum mechanics. Berry realized that this geometry gives quantum particles a kind of memory.
nothing should actually happen
At the time, Berry was studying very simple systems: the quantum states of particles such as neutrons in changing environments. Neutrons have a quantum property called spin, which acts like tiny magnets in particles. This spin causes the north pole to either point up or down, so physicists talk about the neutron “spin up” or “spin down.” The spin of a neutron is affected by an external magnetic field.
Berry used mathematical means to investigate what happens to neutrons when the direction of a magnetic field changes slowly. According to the so-called adiabatic theorem, introduced in the early 20th century, the quantum properties of the particles should not change as a result. That is, the particle’s energy, momentum, mass, and spin remain the same.
If you slowly change the direction of a magnetic field and then bring it back to its original direction, in principle this action won’t actually change anything. “That was the prevailing opinion among physicists for many years anyway,” Berry said. scientific american However, “changes in the phase of the wave function were overlooked.”
One of the strangest phenomena of quantum mechanics is the wave-particle duality. Quantum objects can be imagined as point-like shapes, but they also exhibit wave behavior like water. Phase represents the displacement of a wave through a specific angle. For example, a cosine function is nothing but a sine function with phase shifted.
As Berry realized in his calculations, slow changes in the magnetic field rotate the neutron’s wave function by a certain phase. This means that the particle’s wavefunction tells us what happened in the past, in this case changes in the magnetic field. Furthermore, Berry realized that this step does not occur only in the special case of particles in a magnetic field. Various situations in which a quantum system slowly changes and then returns to its original state leave imprints on the wave function.
Experiments conducted shortly after Berry’s groundbreaking research was published confirmed these ideas. If you are familiar with quantum mechanics, you know that the wave function is not a quantity that can be directly observed. Nevertheless, there are ways to measure the phase shift using a second particle as a reference. In these experiments, physicists allowed two particles, such as neutrons, that had previously been in a varying magnetic field to collide. When the neutrons met, their wave functions interacted.
These features behave like waves in water. When valleys and peaks coincide, they strengthen each other. On the other hand, if they are offset from each other, they can become weaker or disappear completely. These phenomena are known as constructive interference or destructive interference, respectively.
As a result of the experiment, it became clear that Berry’s point was correct. The neutrons are out of phase and interfere destructively. This observation indicated that one of the particles was momentarily present in the changing magnetic field. Even if none of its measurable properties changed directly as a result, its changed wavefunction revealed it.
crooked universe
But how did Berry know that particles undergo a phase change? In fact, such a phase occurs wherever there is curvature. That’s why phase plays an important role in Einstein’s theory of general relativity, the theory he used to explain gravity.
Some experts argue that general relativity is more like geometry than physics. According to this theory, matter bends space-time, and this deformation causes masses to attract each other. This is the phenomenon recognized as gravity. I like to think of this as placing a heavy object on top of a rubber sheet, and the sheet deforms and affects the object. However, this visualization has some weaknesses. In this concept, space-time is two-dimensional, and I’m looking down on it from a three-dimensional world. General relativity, on the other hand, describes the curvature of four-dimensional spacetime without looking from a five-dimensional perspective.
This raises the question: how can we estimate the curvature of an object if we cannot see it from the outside? The phases Berry observed are useful here.
Suppose we want to prove in a complicated way that the Earth is a sphere. To do this, you can walk directly north from where you are, across mountains, valleys, rivers, lakes, and oceans from anywhere in Germany. In this thought experiment, nothing prevents me from following the straight path. When you reach the North Pole, walk sideways like a crab and move to the right without looking back. Walk until you reach the same latitude as your starting point. Then continue along the latitude to the left, without changing direction again, until you return to the starting point. Land in your original location, but instead of facing north as you started, face east. So, while this back and forth didn’t change me as a person (except maybe the physical exertion), it still changed me in one way.
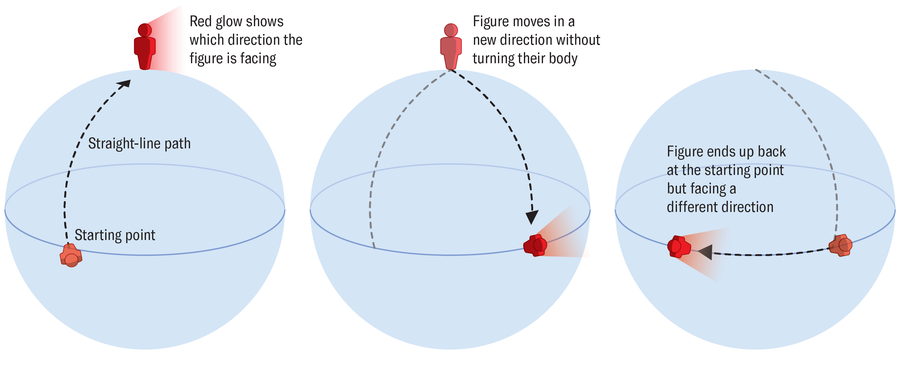
If it had followed the same path on a flat surface, it would have returned to its starting point without turning. But in this thought experiment set on our curved planet, the same thing happens to me as the wave function in Berry’s theory. The wave function takes the phase, angle and shifts it.
The angles I found during my travels depend only on the shape of the Earth. Its value is proportional to the area enclosed by the path. This is called the “geometric phase” because it’s a phase where nothing else affects your speed or whether you take breaks or not.
For mathematicians, this was nothing new at the time Berry published his work. They’ve known about this concept for decades. But no one had applied geometric topology to quantum mechanical processes. The phase of the wave function reveals the geometry of the so-called parameter space. This is an abstract high-dimensional space that combines all the parameters that can affect the wave function (magnetic field, energy, position, velocity, etc.). A short-term change in the direction of the magnetic field (or another parameter) represents a closed curve in this space. Just like the circular path on Earth. This parameter space is usually curved, so it leaves traces in the wavefunction.
“Geometric topology can therefore be seen as the best answer a system can provide to the question, ‘What path did the system take in parameter space?’ ” Berry wrote in 1988: scientific american article. “In this sense, it is a kind of quantum ‘memory’.”
Berry thus revealed a deep connection between quantum systems and geometry, which turned out to be extremely valuable. The Berry phase, named after him, can be used to explain phenomena such as the quantum Hall effect, which occurs in certain solids and raised many questions before Berry’s discovery.
All of this is very exciting. But what is most impressive to me is that Berry used existing mathematical concepts to establish a new field of research: geometric quantum physics. He didn’t need to add anything new to physics or mathematics. Instead, mathematics has made it possible to reveal something completely unexpected in physics.
This article was first published Wissenschaft spectrum Reprinted with permission.

